Propeller hubs and stuff

Ever since my bolt in the connection rod broke, I lost a bit of confidence about certain parts of the engine. The rods in particular, but also the propeller hub. When Sonex has done the most bizarre thing with the rods, and the heads are full of sand, how could I be sure they had not done the same with the hub? I have to find out.

Shrink fit hubs, bolts, bending and fatigue and stuff are typically something we learn during first year when studying mechanical engineering. It's been some decades since I had anything to do with this kind of engineering, but I still got my old books. Instead of speculating about it, I decided to do a text book calculation of AeroVee hub and see how it holds up. It's also fun to refresh some long forgotten knowledge. But since it is old and forgotten, there is no guaranty it is correct... They say building an aircraft takes minimum 2-3 years. If you are an engineer though, multiply that time with 3 :-)

Other engines

It's interesting to look at other engines, just to see how it should be done. The small Continental A-65 and larger are typical examples of how solid aircraft engines should be (the old school).

The Continental has a crankshaft with a tapered hub or a crankshaft integrated hub. It has a large main bearing at the propeller end that also has an integrated thrust bearing. The hub is torqued to the crank with a big nut using 200 lb ft (271 Nm). The Continental can take any propeller. A much more modern engine is the ULPower.

The propeller hub is connected to the crankshaft using splines and a bolt. The thrust bearing is a ball bearing also working as a radial bearing. ULPower can only use modern composite or wooden propellers up to 0.6 kgm² of rotational moment of inertia (same restriction as Rotax 912). The Jabiru has the layout of a Continental, more or less, with a large propeller bearing that also acts as a thrust bearing.

The Jabiru (2200) is restricted to using propellers with a moment of inertia less than 0.2 kgm². Limbach and Sauer use a tapered fit hub, but I have no detailed description, only some drawings from Limbach. They are strong though, up to 160HP and able to work with CS propellers.

Except from the elongated tapered hub, Limbach looks to be almost stock VW, with a smallish propeller bearing. The #3 bearing could be used as a thrust bearing, but I don't know for sure. The L-2000 and L-2400 (80 HP, 2000cc and 87 HP, 2400 cc) is preferred running with a 3 bladed Hoffmann HO-V62 CS prop with a moment of inertia of 0.5 kgm². The Revmaster has a configuration approximately like the Continental.

The hub is tapered (3 degrees) and is held by a left hand 3/4" bolt torqued to 150 lb ft. The #3 bearing is modified to also work as a thrust bearing. The #4 bearing, on the VW it is only 15 mm long, is replaced with a new bearing of what looks to be 40 mm. Revmaster can use similar propellers as Limbach/Sauer. Great Plains and Hummel use the "Force One" hub from Great Plains, which is a similar concept to the Revmaster hub. This also has a 3 degree taper and is held in place by a M12 or M20 bolt with fine threads, torqued to 60 or 80 lb ft (81-108 Nm)

The thrust bearing on the Great Plains engine is the original #1 bearing (at the back). Only "light wooden propellers" shall be used. The AeroVee has a totally different configuration. It uses the original 15 mm #4 bearing and the #1 bearing as a thrust bearing. The hub is shrink fit and also held in place by a M12 bolt. With the AeroVee 2.1 came a slightly larger diameter hub interface with a smaller diameter bolt (M12 instead of M20 apparently). The old 2.0 and the new 2.1 crankshaft can be seen below.

The result of this new crankshaft is much more material where it is needed, still the axial length of the shrink fit connection is considerably shorter than the taper fit of the custom made Sauer/Limbach crankshafts. Aerovee is only approved for "light wooden propellers".

The Continental is a old school design. Strong and simple and impossible to beat in anything except weight. It only has 3 bearings + thrust bearing, but the #3 bearing is very large. The Jabiru has the same general layout with a large #3 (actually #5 for the 2200) bearing being thrust and radial. In contrast with the Continental, it can only take propellers up to 0.2 kgm². The ULPower looks skinny, almost flimsy with that small ball bearing, yet it can take propellers up to 0.6 kgm². The same goes for Limbach/Sauer using what looks to be stock #4 VW bearings, and they regularly use heavy CS props (max 0.5-0.6 kgm²). The Great Plains with that huge Force One hub, can still only take "light wooden propellers". Limbach and Sauer has sold more than 7000 engines altogether, most of them with CS/manual variable pitch props on motorgliders, and I have not heard of any broken cranks. What is going on?

Well, in my opinion, except for the Continental that is built like a tank, the most elegant solutions, by far, are Sauer/Limbach and ULPower. ULPower being the number one. The AeroVee 2.1 is also rather elegant, but the shrink fit hub, although strong, is an unnecessary complication that leaves room for manufacturing and mounting errors, and it is practically impossible to take it apart without weakening it or destroying it altogether. Shrink fit hubs exists in two main variants: permanent and removable. There exist dozens of different removable shrink fit hubs; mechanical, hydraulic, clamp on etc, and the AeroVee is not one of them, it is the permanent variant. The Force One and Revmaster are OK, the hub/crank connection is a tapered one and should be very strong. The reason for the huge bearing completely eludes though. I see the large bearing more as a result of being able to make a good and strong tapered hub with a stock VW crankshaft with a short "nose". The huge bearing has no real mission in there as I see it. Look at the crankshaft of the Continental, it is nothing but a thin bended tube. The counter weighted crank shaft of the VW is massive in comparison, also compared with ULPower and Jabiru. The shaft is not only strong, it is also immensely stiff. The Continental needs a long prop bearing to stiffen it up to prevent too much angle on the thrust bearing, but this could also have been done with two bearings, one large main bearing and one smaller further out, which by accident happens to be the stock VW solution and by design the ULPower solution (even though the ULPower has a different configuration altogether). The VW has three massive (relative speaking) main bearings supporting an even more massive crankshaft, and a thrust bearing at the aft end.

A small peculiarity is the difference between ULPower and AeroVee on one side against the others. This is particularly visible for the ULPower. A spline connection is only designed to take torsion. Bending is taken by the bolt pressing the inner face of the hub against the end face of the shaft, axial forces are also taken by this bolt. The exact same thing is in fact done on the AeroVee hub, thus the shrink fit interface only needs to take torsion. In practice, due to stresses and strains there will be a combination of everything everywhere, but the principle remains and is valid. For a tapered hub, all the forces has to go through the tapered interface, this also includes the bending moments, and to a large extent the axial forces.  

Ultimately what makes a good design is one that proves it will hold together over time, and also is practical, serviceable. Continental certainly has proven itself, and so has Limbach/Sauer, Revmaster and Great Plains. The Jabiru has had several problems, and so has AeroVee 2.0. ULPower is still new, but it certainly is serviceable. The question remains, will the AeroVee 2.1 hub hold together? That is what I am trying to find out.

Engine Torque

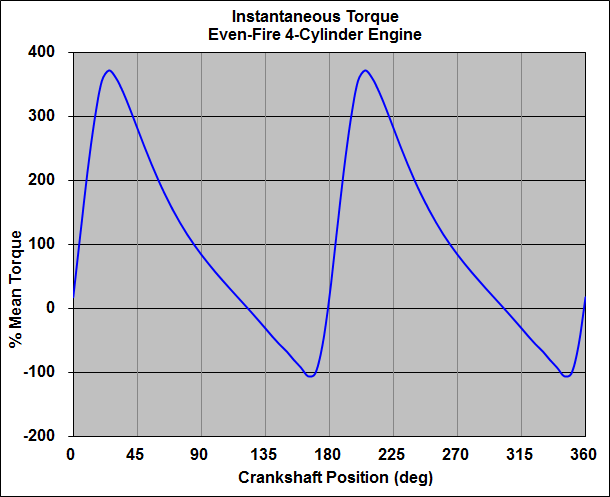
The main force working all the time the engine runs is the torque. This torque varies with each firing of the cylinders. EPI has some good info about this, and the torque at the output of the shaft looks like this for a 4 cylinder, 4 stroke engine:

When the engine runs at max torque at 3000 rpm, 6000 of these pulses occurs each minute, it is obvious the main damaging factor is fatigue. After only 28 hours run, 10⁷ cycles has occurred. 10⁷ cycles is often considered to be the maximum if the part shall not break because of fatigue. This means an hub/shaft has to be sized to withstand an indefinite numbers of cycles. To be able to withstand an indefinite number of cycles, the amplitudes has to be lower than a certain max of the material used.

The mean torque for the AeroVee is 127 lb feet, or 172 Nm. The torque on the shaft is this mean +- the variation, +375% -100% according to EPI when looking at the graph. From a fatigue point of view the torque will consist of the algebraic mean using only the peaks. That is, the mean torque is 236.5 Nm, plus a varying torque of +- 408.5 Nm, or 236.5 Nm +- 408.5 Nm.

Flywheel

The torque pulses above could be hard on the propeller hub. Luckily the VW engine also has a flywheel. The purpose of the flywheel is to even out these pulses (as well as doing more practical things as helping with ignition and the starter). A flywheel absorbs energy when the speed increases and releases energy when the speed decreases. The energy stored in a rotating flywheel is:

E_k = 1/2 * I_p * omega²

I_p is the polar moment of inertia. The energy in the flywheel is proportional to the square of the rotating speed, so the faster the engine runs, the smaller the torque pulses becomes (at least in angular displacement and angular velocity), because more energy is stored in the flywheel compared to the energy in the pulses themselves (the torque pulses are more or less constant at WOT, at least up to some RPM). The crankshaft itself also works as a flywheel, as do all rotating and moving masses in the engine. For the AeroVee, the crankshaft is particularly heavy with the large counterweights. The total flywheel of the engine is therefore the flywheel itself + the crankshaft and rods. The graph from EPI is probably realistic for an engine with a very light crank shaft, but not very realistic for the AeroVee with a heavy crankshaft and a flywheel, not if the torque pulses at the propeller hub is of interest.

What is happening is the surplus torque (the torque above the 100% mean in the graph), is used to accelerate the rotating masses, and the torque below the 100% line is used to decelerate the rotating masses back again. If somehow the rotating speed was held constant, for instance with a very heavy and large propeller, then the flywheel would not "work" and the full torque amplitudes would go through the propeller hub. In effect, variations in torque at the hub is traded for variations in engine speed. A purpose designed aircraft engine (Lycoming or any other heavy duty engine, not Jabiru or Rotax btw) is designed to use heavy metal propellers as the only flywheel, thus the propeller hub is designed accordingly to withstand the pulses from the engine, almost regardless of the weight of the propeller.

Essentially there are three flywheels. The flywheel itself and the crankshaft, and the propeller. A look at the engine and the propeller separately will give variation in rotational speed for both of them as:

delta_omega = (E_max - E_min)/(I_p * omega_mean)

omega_mean is the RPM (in rad/s) of interest as input. The difficult part are the E_max, E_min and the I_p. The I_p of the crankshaft and the flywheel are calculated as a cylinder and a disk respectively:

I_p = 1/2 * m * r²

The flywheel: m = 4 kg, r = 0.115 m. The crankshaft (+ rods): m = 15 kg, r = 0.065 m. The total I_p is the sum of both, and is: I_p_e = 0.06 kgm²

The Ip of the propeller is much more difficult, and the only practical way to find it, is to measure it by doing a pendulum experiment. I did just that, and the stock Sensenich propeller has a moment of inertia of 0.165 kgm².

This means the propeller is a factor 2.73 "heavier" than the total flywheel and the engine. For the propeller hub, more Ip on the engine side is better, and less Ip on the propeller side is better.

To find the E_max - E_min it is enough to calculate the red area in the figure above. This can be done since the angle at the start of the red area correspond to the minimum input of surplus energy (the shaft has just come back from a trip into the less than mean torque), and the angle at the end of the red area correspond to the maximum input of surplus energy. It's a bit fiddly to do the exact integration, and since the graph only is a general representation of a 4 stroke 4 cylinder engine, the area is simply calculated by approximating two triangles. One triangle from 8 to 25 degrees, and one from 25 to 80 degrees. This must be done using radians.

A = 1/2 * ((645-172)*(0.436-0.140) + (645-172)*(1.396-0.436)) = 297 J

Now delta_omega can be calculated, and is shown in the graph below.

The graph shows that when the RPM is free to oscillate, the osculations will diminish with increasing mean RPM. Thus the pulses from the engine to the prop hub will decrease. The propeller itself will also become "stiffer", but in the same ratio as the flywheel. The net effect is the pulses to the hub becomes smaller and they are also reduced by a constant factor given by the Ip of the prop and the Ip of the flywheel. The Ip of the prop being 2.73 times larger than the Ip of the engine. So, with a propeller of an Ip of 0.165 kgm², the torque pulses on the hub are reduced by a factor 1/2.73 or reduced to 63%. 63% of the rotating masses are in the propeller, while 37% are in the engine and flywheel.

The effect on the hub of the reduced oscillations due to increased RPM cannot really be predicted in a meaningful way without also taking into account the stresses and strains of the whole engine. The simplest approximation that can be done is to see the the propeller and the engine as two masses connected with a flexible shaft. This will be a much more complex analysis with a lot more factors to consider, and is not really needed to get to the conclusion as shown later. This torque pulses are still there, the angular acceleration is constant, but their duration becomes shorter and shorter with increasing RPM, and so will also the angular displacement of the shaft.

So, the rotating masses of the engine and the light weight propeller will reduce the torque pulses from 236.5 +- 408.5 Nm to 236.5 +- 257.4 Nm.

Shrink fit hub

Static analysis

The shrink fit itself must be calculated to find the needed shrinkage to assure enough friction between the hub and the shaft is applied without too much tension. This is done using the max torque. The max torque is 494 Nm (when the flywheels have done their job). The dimensions of the hub and shaft is needed also.

These dimensions are (or very close):
L = 25 mm, the length of the shaft/hub shrink connection.
d = 38 mm, the diameter of the shaft
di = 12 mm, the diameter of the hole for the bolt in the shaft.
D = 55 mm, the diameter of the propeller hub to be shrunk onto the shaft

Now the pressure, or tension, needed can be calculated. This also include the friction coefficient for for steel-steel shrink fit connection with varying load, ny (greec letter). This ny is 0.10

The pressure needed is p_min = T*2/(ny*Pi*d²*L) = 87.1 MPa

The delta, or diameter difference can be calculated now. This involves calculating two more coefficient, alpha_a and alpha_n. These coefficient is are geometric relations incorporating the modulus of elasticity and the poisson ratio. The delta is delta = p_min*(alpha_n + alpha_a) = 0.034 mm. For a 38 mm shaft this is within a so called H7/r7 interference fit.

With this fit, the tension on the shaft is max -193 MPa (pressure) in tangential direction. This tension is at di, the 12 mm hole for the bolt.

This is the minimum delta needed to obtain the torque. The safety factor lies within the friction coefficient, and is about 1.5-2.0. The safety factor is needed to allow for slight ovalisation, unequal cooling, contaminations and so on. 193 MPa is not that much for axle steel, not in pressure. The max tension on the hub is only 87.1 MPa (in tension). CrMo axle steel typically can withstand up 800-1100 MPa in tension before it breaks.

It is of great importance that the delta actually is at least this minimum, any smaller and the hub will not hold, it will start to slip and break rather quickly. The manual say to heat the hub to 450 degree f, or about 200 deg C. This is about 175 K above ambient. This causes a the hole to grow about 0.08 mm.

All in all, consider accurate production of parts and correct procedure when mounting the hub, the shrink fit hub should be all OK from a static point of view.

Fatigue analysis

This is a lot more tricky because the exact material must be known. Assuming nitrided AISI 4340 steel, this will have a fatigue limit tensions of approximately +-380 MPa in tension and +-470 MPa in bending and an Rm of 880 MPa for a 40 mm shaft (according to my books). There is no fatigue limit in torque, because an equivalent tension is calculated.

The torque tension (tau) is T*d/Ip where Ip is the polar moment of area of the shaft with the 12 mm hole.
tau_mean = 44.3 MPa
tau_amp = 48.3 MPa

The hub will stay put, as calculated above, but the forces has to go through the "slice" at the inner edge of the hub. Here there is lots of strangeness. First, the fatigue properties is weakened simply by the shrink fit itself. This factor is about 2.0-2.6 in bending and 1.7 in torsion. The woodruff key will weaken it by a factor of 3.4 in bending and 2.0 in torsion. Then there is a diameter difference in the shaft just inside the hub. This will also be a factor 1.5 - 2.0. These factors are a bit fuzzy, this is because fatigue is a statistical phenomenon, and the statistics gets worse when there are oddities in the design. One cannot possibly foresee every possibly oddity, and therefore these "standard" factors. To get more accuracy a full 3D FEA must be done. Here there is bending and there is torsion, and the largest (worst case) factor is chosen, which is 3.4

The fatigue is a result of mean tension + oscillating tension. The mean tension is small here, but not zero, due to the bolt. How exactly the bolt will affect this, I don't know, but the effect should be small if it is not over torqued. The mean torque is not part of the equation, it is unimportant as shown by experiments (bending and linear tension is important though). So, the only important factor here is the oscillating torque. The factor above accounts for variations in stress and shear etc.

The sensitivity (eta) for these factors are approximately 0.7 Then the main factor, Kfs, is calculated as:
Kfs = 1 + eta*(Kts -1)
The equivalent amplitude, will in this case be:
sigma_ea = sqrt(3*(Kfs*tau_amp)²) = sqrt(3)*tau_amp*Kfs = 83.6*Kfs MPa

Then there is the question of fatigue amplitude of the material. Will it fatigue in axial tension, or in bending? Obviously in torsion, but material data only gives bending and axial tension. Lets say bending, +-470 MPa. This number also has to be adjusted by a dimension factor of 0.81 due to small size, and 0.95 (polished). The fatigue amplitude becomes 0.85*0.95*470 = +- 380 MPa.

The end result is the security against fatigue : n_u = 380/(83.6*Kfs)

Then consider the largest single Kts of 3.4 Then n_u = 1.69 which is a good safety margin. If the tension factor was used then n_u = 1.37. Let say it is somewhere in between, which is 1.5.

So, the shrink fit hub is indeed strong enough in both static and fatigue analysis. But again, correct dimensions and procedure is crucial.

Bending

There is also bending involved due to gyroscopic forces. At first look the number of "bends" cannot possibly be anywhere near the number of torsional pulses (6000 each minute at 3000 RPM). However, it turns out this is a much more complex phenomenon, and the number of bending pulses may indeed be 12000 each minute, and may do this with an amplitude comparable to the torque pulses.

The reason for this, is the gyroscopic forces acting on the propeller. Gyroscopic forces are functions of the polar moment of inertia in x, y and z direction. Consider x runs through axis of rotation, y runs along a blade, and z runs perpendicular to a blade. Now the moment in x is the Ip as above. The moment in z (causing the propeller to cartwheel) will also be almost identical to Ip. But the moment in y, causing the propeller to rotate around the blade axis will be very small compared with the others. That is, a 2 bladed propeller is not symmetric in Iy and Iz. With 3 or more blades, the propeller suddenly becomes symmetric in Iy and Iz and behaves like a disk, a 2 bladed propeller does not. All this is explained in detail in the book Gyrodynamics and Its Engineering Applications by Arnold and Maunder.

The result for a two bladed propeller becomes:

Tx = 0
Ty = Ip * w_p * w_z + (Iy - Iz)*w_p*(w_z*cos(2*w_p*t)-w_y*sin(2*w_p*t))
Tz = -Ip * w_p * w_y + (Iy - Iz)*w_p*(w_y*cos(2*w_p*t)+w_z*sin(2*w_p*t))

Here w_p is the rotational speed of the propeller and w_y and w_z is the pitch and yaw rotational speed of the aircraft. t is time. Iy and Iz is the propeller moment of inertia about the y and z axis. For a 3 or more bladed propeller, Iy - Iz = 0, and the torque moment simply becomes:

Tx = 0
Ty = Ip * w_p * w_z
Tz = -Ip * w_p * w_y

For instance in a loop (considering 2 bladed propeller), w_z = 0, and Iy is so small it is practically equal to zero, and that Iz = Ip:

Ty = - Ip*w_p*w_y*sin(2*w_p*t) = +- Ip*w_p*w_y*sin
Tz = - Ip*w_p*w_y*( 1 + cos(2*w_p*t)) = Ip*w_p*w_y*sin +- Ip*w_p*w_y*sin

This means the gyroscopic moment from the propeller oscillates about the y axis with the amplitude Ip*w_p*w_y going back and forth 2 times each propeller rotation. In addition it has a constant moment about the z axis of Ip*w_p*w_y with an oscillation on top of this so the minimum is zero and the maximum is 2 times Ip*w_p*w_y, and it happens two times for each propeller revolution.

In contrast, a 3 bladed (or more) propeller has a steady and nice torque with an amplitude of Ip * w_p * w_y. Not only is the torque from the 2 bladed prop twice as large, it is also oscillating two times each propeller revolution and it is oscillating in two directions.

A loop takes minimum 5 seconds perhaps (a very tight one), omega_a = 2*pi/5, omega_p is 335 rad/s (3200 rpm). Ip is as before. The bending moment from the propeller acting on the shaft becomes:

Ty = +- 69.5 Nm
Tz = 69.5 Nm +- 69.5 Nm (min = 0, max = 139 Nm)

Obviously this will add to the fatigue calculations and lower the safety against fatigue, but by how much? It is impossible to say. 1 hour with straight and level flying does nothing, while 1 hour with acro does a lot. The corresponding stresses becomes:

sigma_y = +- 11 MPa
sigma_z = 11 MPa +- 11 Mpa

Still, this won't hardly do any effect on the safety against fatigue. The sigma_ea when including bending becomes one of:

sigma_ea = sqrt((Kfb*sigma_y)² + 3*(Kfs*tau_amp)²)

The addition from bending is not even 1%, so the gyroscopic moment is completely insignificant relative the torque pulses regarding fatigue even if looping all day long, year after year.

The number 4 bearing

So what about the bearing? This depends entirely on how much the shaft moves to one side. Max gyroscopic torque is 139 Nm in a loop. The length of 40 mm shaft is about 50 mm. Max movement when there is a torque moment at the end becomes:

u_max = T*L² / (2EI) = 6.6 micrometer (1 micrometer = 1/1000 millimeter). Consider it will move some more due to the main bearing also moves, let say 12 micrometer. It is important to note the gyroscopic moment from the propeller is one of pure bending moment, it does not act as a side force pushing the propeller hub to the side.

delta_r in a bearing can vary a lot according to several variables. The shaft is 40 mm shaft and the length of the bearing is 15 mm. 15/40 = 0.375. This number certainly could be longer, the optimum is about 1.0, but this is what it is. A typical minimum film thickness d = 40 mm, and rpm of 3500 and below is 5 micrometer. From my books, this means an "epsilon" of about 0.8, and a delta_r = 5*10⁻⁶/(1-0.8) = 0.025 mm = 25 micrometer. So the conclusion is that this bearing will not take much load from the gyroscopic forces at all, simply because the gyroscopic forces aren't that large with a light wooden propeller. The gyroscopic forces will hardly put the bearing at work, the crankshaft itself is so massive that it's role here is purely as a secondary support.

The conclusion is the stock #4 bearing with a beefy crank shaft is OK. This is hardly news, Limbach has figured this out ages ago.

Summary shrink fit

The shrink fit hub with the woodruff key and strangeness is not an optimum design. Dismantling it must be extremely complicated, and IMO impossible to do without rendering it unusable. But as far as I can see it is more than strong enough for all forces in action. The largest force is the torque pulses from the engine. These pulses can cause fatigue. With a light wooden propeller and the rotational inertia of the engine and flywheel, the design is still well within limits. It will go on for ever and ever without breaking.

The static forces, the shrink pressure needed to hold the torque is also just fine.

The gyroscopic forces, although peculiar with a 2 bladed prop, will not affect the fatigue with as much as 1%, even if the aircraft is continuous looping. The bending moment is not large enough for the number 4 bearing to even start "working".

All in all, I can't find anything structurally "wrong" with the AeroVee 2.1 hub considering the material quality is OK. If it is put together correct and used with a light wooden propeller, it should hold for ages and ages. The only thing, it is a use and throw away piece. If the bearings or cogwheel between the crank gets worn, the only option is to get a new crank and hub. Maybe some fancy shock heating process will do it, but how? With this is mind, the well proven Force One prob hub that can be re-build an indefinite number of times seems like a much better option to start with.

Update on the #4 bearing

Having thought a bit more about the #4 bearing along with some new info, there are more considerations. Propeller tracking is one, the actual bearing dimensions (inner diameter) is another one and the material and production method of the crankshaft is a third. Lots of more information and experience can be read here and here. A YouTube movie of an actual crank break in Sweden on an Aerovee not long ago can be seen below (at approximately 5:00).

Exactly why and how is a bit difficult to deduce from that video. But what can be seen is fatigue cracks due to torsional tensions. The cracks at 45 degree angles leaves no doubt about that. Too advanced timing, slightly high compression, running on mogas, could all cause detonation that will cause torsional forces way above the figure from EPI (see above). The crank appears to be a 2.0 version, the old one. I have been in contact with Mr Chiriac, and he has sold all the parts (at 1/4 of the price) and bought a Rotax 912 ULS.

The main concern regarding that video though, is it appears to have started at the wudruff key at the cam gear. It has nothing to do with the hub. The reasons may be the same as above, but bending is also a major factor now. Bending from propeller tracking in particular. A correctly fitted radial bearing would carry bending loads better, if this is a concern. I had to look at my bearing. Ideally the clearance should be 25 micrometer, but it is a lot more than that, more than 100 micrometer at least. See video below. All wrong. This is the typical way it breaks according to history. It starts at the key in the hub, grows back to the key in the timing gear and breaks there.

This means the #4 bearing does nothing. It serves no purpose, the clearance is too large to create a working film. With a light wooden propeller this really should be no concern though. The crankshaft should be strong enough as it is, even without the bearing. The problem is, it is not, or was not. It is therefore tempting to reach a conclusion the problem is metallurgical. The crankshaft is poor quality steel. I have a hard time believing this would happen to good quality forged 4340 steel, detonation should break rods or pistons before the krankshaft. I have asked Aeroconversion about steel and method, no answer so far.

Update 2

Tried to measure the clearance. It if very hard to do, but a "blade meter" (or whatever it is called in English) gives some idea. A 0.05 mm blade enters, a 0.1 mm does not. This means the radial clearance is larger than 0.025 mm but smaller than 0.05 mm. This does not look bad at all if enough oil enters into it. Got an answer from Sonex about the crankshaft, nothing from Aeroconversions as of yet (They are the same company, sort of, but still).

4340 Forged –and- Nitrided Steel.

The crank is made specifically for us by the only major VW racing crankshaft supplier in the world. We do not reveal our specific sources for custom AeroVee parts.
Sonex Aircraft Tech Support


One can only speculate about their secrecy, but 4340 forged - good. Nitrided - 4340 must be hardened somehow on the surface.

In the end. The crankshaft is made of 4340 forged and nitrided. It is made by the only major VW racing crankshaft supplier in the world (whoever that is, presumably a quality stamp?)  The nose of the crank is re-designed with much more material. I have calculated the fatigue myself, no problem. Bending due to gyroscopic forces, hardly an issue whatsoever with the light wooden propeller. No reported breaks of the 2.1 crank. Time to build that engine and move on.